
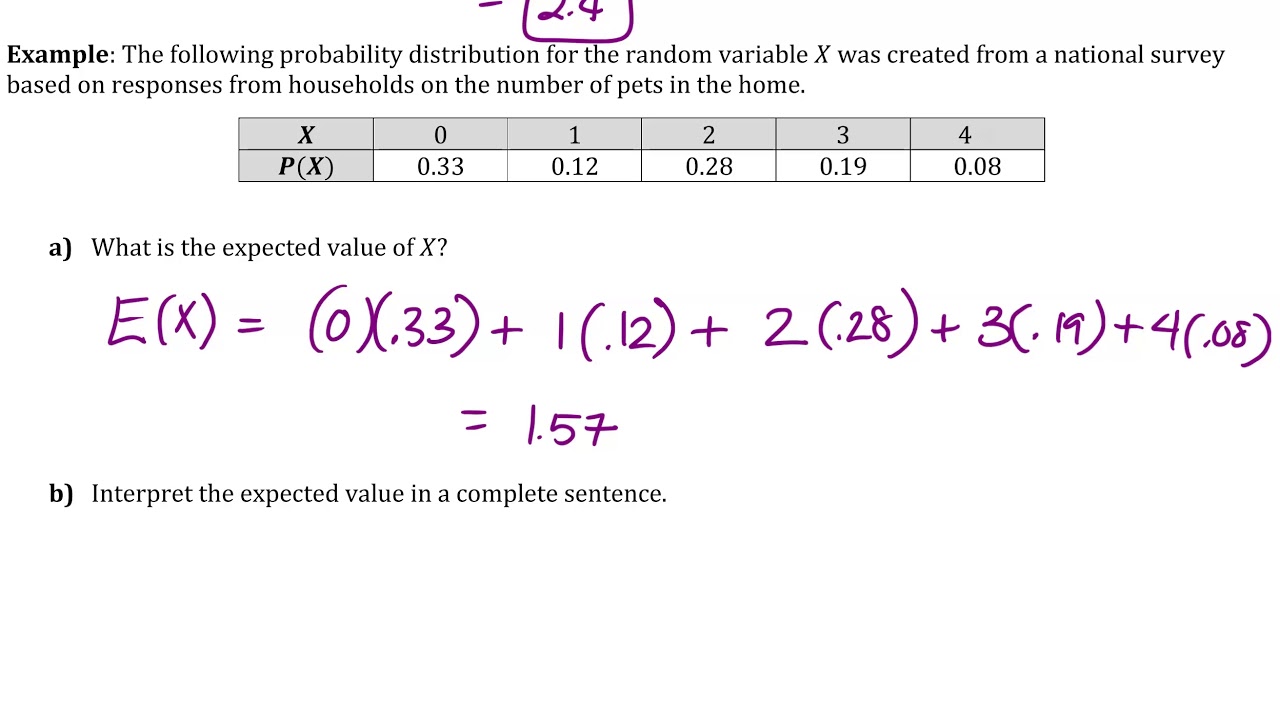
You do this because you can't do the final step of the anova, comparing the Y intercepts, if the slopes are significantly different from each other. Next, you see whether the slopes are significantly different. exclamationis is Ŷ=3.75 X−11.0, and the line for O. In the cricket example, the regression line for O. The first step in performing an ancova is to compute each regression line. I have no idea how sensitive it is to deviations from these assumptions. AssumptionsĪncova makes the same assumptions as linear regression: normality and homoscedasticity of Y for each value of X, and independence.
exclamationis), the adjusted means give you some idea of how big this difference is compared to the mean. The Y intercepts are -7.2 and -17.3 pulses per second, respectively while the difference is the same (10.1 more pulses per second in O. niveus these are the predicted values at the mean temperature of all observations, 23.8 ☌. For the cricket data, the adjusted means are 78.4 pulses per second for O. Stating the null hypothesis in terms of Y intercepts makes it easier to understand that you're testing null hypotheses about the two parts of the regression equations stating it in terms of adjusted means may make it easier to get a feel for the relative size of the difference. Because the regression lines you use for estimating the adjusted mean are parallel (have the same slope), the difference in adjusted means is equal to the difference in Y intercepts. The adjusted mean for a group is the predicted Y variable for that group, at the mean X variable for all the groups combined. Some people define the second null hypothesis of ancova to be that the adjusted means (also known as least-squares means) of the groups are the same. If you accept the null hypothesis that the regression lines are parallel, you test the second null hypothesis: that the Y intercepts of the regression lines ( a) are all the same. The first null hypothesis of ancova is that the slopes of the regression lines ( b) are all equal in other words, that the regression lines are parallel to each other. Remember that the equation of a regression line takes the form Ŷ= a+ bX, where a is the Y intercept and b is the slope. You test two null hypotheses in an ancova. temperature in two cricket species, Oecanthus exclamationis (solid circles and line) and O. exclamationis would have a higher pulse rate at any temperature. exclamationis is higher than the line for O. You can control for temperature with ancova, which will tell you whether the regression line for O.

niveus at some temperatures but not others. exclamationis might have a higher rate than O. exclamationis measurements was 3.6 ☌ higher than for O. This confounding variable means that you'd have to worry that any difference in mean pulse rate was caused by a difference in the temperatures at which you measured pulse rate, as the average temperature for the O. However, you can see from the graph that pulse rate is highly associated with temperature. niveus, and the difference is highly significant ( two-sample t–test, P=2×10 −5). If you ignore the temperatures and just compare the mean pulse rates, O. He measured the pulse rate of the crickets at a variety of temperatures: O. Walker (1962) wanted to know whether the chirps of the crickets Oecanthus exclamationis and Oecanthus niveus had different pulse rates. Each wingstroke by a cricket produces a pulse of song, and females may use the number of pulses per second to identify males of the correct species. For example, Walker (1962) studied the mating songs of male tree crickets. It is a way of comparing the Y variable among groups while statistically controlling for variation in Y caused by variation in the X variable. The purpose of ancova is to compare two or more linear regression lines. The nominal variable divides the regressions into two or more sets. Use analysis of covariance (ancova) when you have two measurement variables and one nominal variable. Use analysis of covariance (ancova) when you want to compare two or more regression lines to each other ancova will tell you whether the regression lines are different from each other in either slope or intercept.


 0 kommentar(er)
0 kommentar(er)
